

#GEOMETRIC SEQUENCE EQUATION HOW TO#
To learn how to find the nth term in a geometric progression, see the example ahead.įind the 8th term for a sequence. One way is to use the geometric sequences calculator. How to find the nth value in a geometric sequence? We use a formula to find any number value in a geometric sequence. “Such a sequence in which the difference (d) between the two consecutive terms is a ratio (r)”Įach new term is found by multiplying the preceding term with this ratio. For example:Īll of the values in this sequence differ from their previous value by -2. Multiply the resultant by the first term, a. The number being multiplied each time is constant (. To find the sum of a geometric sequence: Calculate the common ratio, r raised to the power n. It means that each term is different from its previous value in the same way as the term next to it is from itself. Geometric sequences follow a pattern of multiplying a fixed amount (not zero) from one term to the next.
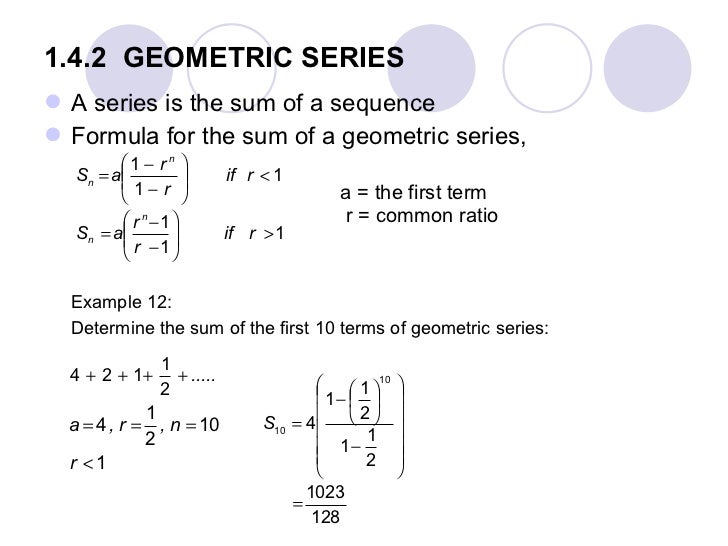
In general, a sequence is a set of integers that go on with a flow. This tool gives the answer within a second and you can see all of the steps that are required to solve for the value, yourself. A geometric sequence is a sequence of numbers in which each new term (except for the first term) is calculated by multiplying the previous term by a constant value called the constant ratio ((r)). You can enter any digit e.g 7, 100 e.t.c and it will find that number of value. The common ratio can be found by dividing any term in the sequence by the previous term. The constant ratio between two consecutive terms is called the common ratio. It uses the first term and the ratio of the progression to calculate the answer. A geometric sequence is a sequence in which the ratio between any two consecutive terms is a constant. The geometric progression calculator finds any value in a sequence. A geometric sequence, or geometric progression, is a sequence of numbers where each successive number is the product of the previous number and some constant r.


 0 kommentar(er)
0 kommentar(er)
